Convert the given equation both to cylindrical and to spherical coordinates. x^2+y^2=2 x

Convert the given equation both to cylindrical and to spherical coordinates. z=x^2-y^2Подробнее
Convert the given equation both to cylindrical and to spherical coordinates. x^2+y^2=2 xПодробнее

Convert the given equation both to cylindrical and to spherical coordinates. z=x^2-y^2Подробнее

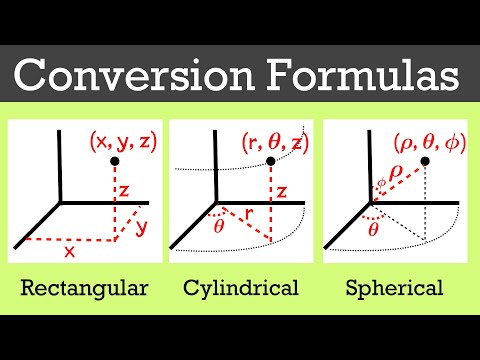
Rectangular, cylindrical, and spherical coordinates (introduction & conversion)Подробнее
Calculus 3: Triple Integrals in Spherical Coordinates (Video #25) | Math with Professor VПодробнее
